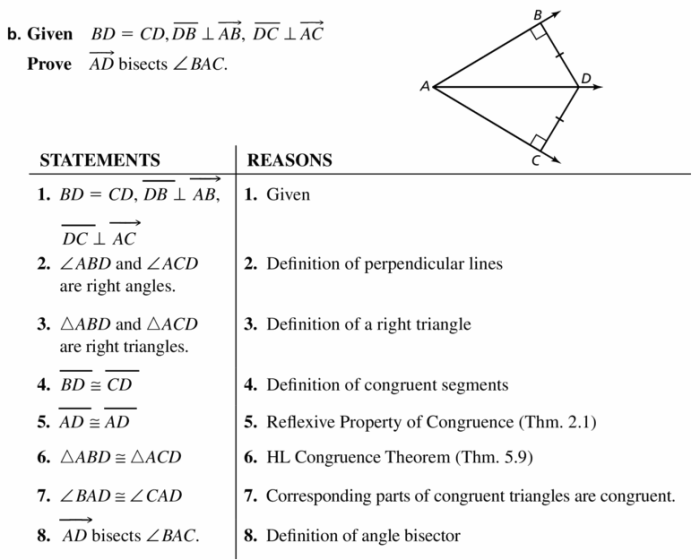
Embark on an enlightening journey with Big Ideas Math Geometry 12.2 answers, where the intricacies of circles unfold. This chapter delves into the fundamental concepts of circles, unraveling their properties and applications in a captivating manner.
Prepare to master the definitions of circles, explore the relationship between radius and circumference, and unravel the mysteries of angle relationships within circles. Moreover, you will discover the properties of tangents and delve into real-world applications of circle properties.
1. Introduction to Big Ideas Math Geometry 12.2
Big Ideas Math Geometry 12.2 is a chapter that focuses on the study of circles and their properties. Circles are fundamental geometric shapes with numerous applications in various fields. This chapter provides a comprehensive understanding of the key concepts and theorems related to circles, enabling students to develop problem-solving skills and apply their knowledge to real-world situations.
The chapter covers essential topics such as defining circles and their key elements, exploring the relationship between the radius and circumference of a circle, and understanding the properties of inscribed angles, central angles, and intercepted arcs. Additionally, it delves into the concept of tangents to circles and their properties, providing students with a deeper understanding of the geometric relationships involved.
2. Properties of Circles
A circle is a closed, two-dimensional figure consisting of all points equidistant from a fixed point called the center. Key elements of a circle include the radius, which is the distance from the center to any point on the circle, and the diameter, which is the distance across the circle through the center.
One of the fundamental properties of circles is the relationship between the radius and circumference. The circumference of a circle is the distance around the circle, and it is directly proportional to the radius. The formula for calculating the circumference of a circle is C = 2πr, where C represents the circumference, r represents the radius, and π (pi) is a mathematical constant approximately equal to 3.14.
3. Angle Relationships in Circles
Circles have specific angle relationships that are important to understand. Inscribed angles are angles whose vertices lie on the circle and whose sides are chords of the circle. Central angles are angles whose vertices are at the center of the circle and whose sides are radii of the circle.
A key theorem in this context is the Inscribed Angle Theorem, which states that the measure of an inscribed angle is half the measure of its intercepted arc. This theorem allows for the determination of angle measures within circles.
4. Tangents to Circles
A tangent to a circle is a straight line that intersects the circle at exactly one point. The point of intersection is called the point of tangency. One of the properties of tangents is that they are perpendicular to the radius of the circle at the point of tangency.
Another important property is that the length of a tangent from a point outside the circle to the point of tangency is equal to the distance from the point to the center of the circle minus the radius.
5. Applications of Circle Properties, Big ideas math geometry 12.2 answers
The properties of circles have numerous applications in various fields. In engineering, circles are used in the design of gears, pulleys, and other mechanical components. In architecture, circles are used to create arches, domes, and other structural elements. In art and design, circles are used to create patterns, logos, and other visual elements.
Understanding the properties of circles is essential for solving problems in these and many other fields. By applying the theorems and concepts learned in this chapter, individuals can effectively design, create, and analyze objects and structures that involve circles.
Expert Answers: Big Ideas Math Geometry 12.2 Answers
What is the formula for calculating the circumference of a circle?
C = 2πr
How do you find the measure of an angle formed by two chords?
The measure of the angle is half the sum of the measures of the intercepted arcs.
What is the relationship between the radius of a circle and the length of a tangent?
The length of a tangent from a point outside the circle is equal to the radius of the circle.